Thursday, May 6, 2010
Amp & SHift
showing how to move the the equation w/ the amp. & shift
Wednesday, May 5, 2010
final!!!!!!!
sin A
a = sin B
b = sin C
c
This can also be interpreted as three equations:
sin B
b = sin C
c , sin A
a = sin C
c , and sin A
a = sin B
b
The law of cosines
c2 = a2 + b2 – 2ab cos C
There are two other versions of the law of cosines,
a2 = b2 + c2 – 2bc cos A and b2 = a2 + c2 – 2ac cos B.
n a circle whose radius is 10 cm, a central angle θ intercepts an arc of 8 cm.
a) What is the radian measure of that angle?
Answer. According to the definition:
θ = s
r = 8
10 = .8
b) At that same central angle θ, what is the arc length if the radius is
b) 5 cm?
Answer. For a given central angle, the ratio of arc to radius is the same. 5 is half of 10. Therefore the arc length will be half of 8: 4cm.
Converting radians and degrees
Convert 200° into radian measure:
200° (Π/180°) = 200/180Π radians or 3.49 radians
Convert 1.4 radians into degrees: 1.4 (180°/Π) = 80.2 °
http://www.math.uakron.edu/~tprice/Trig/Graphs.pdf Great powerpoint on sine and cosines waves.
Scribe Post review 5.1-5.3 Nigel.
The ambiguous case
The so-called ambiguous case arises from the fact that an acute angle and an obtuse angle have the same sine. If we had to solve
sin x = ½,
for example, we would have
x = 45° or x = 135°.
(Topic 6, Example 1.)
In the following example, we will see how this ambiguity could arise.
In triangle ABC, angle A = 30°, side a = 1.5 cm, and side b = 2 cm. Let us use the law of sines to find angle B.
sin B
sin 30° = 2
1.5
Since sin 30° = ½ (Topic 7, Example 2),
sin B = ½· 20
15
= 10
15
= 2
3
.666
On inspecting the Table for the angle whose sine is closest to .666, we find
B42°.
But the sine of an angle is equal to the sine of its supplement. That is, .666 is also the sine of 180° − 42° = 138°.
This problem has two solutions. Not only is angle CBA a solution,
but so is angle CB'A, which is the supplement of angle CBA. (We can see that it is the supplement by looking at the isosceles triangle CB'B; angle CB'A is the supplement of angle CB'B, which is equal to angle CBA.)
Given two sides of a triangle a, b, then, and the acute angle opposite one of them, say angle A, under what conditions will the triangle have two solutions, only one solution, or no solution?
Let us first consider the case a < b. Upon applying the law of sines, we arrive at this equation:
1) sin B = sin A· b
a .
Now, since h
b = sin A , where h is the height of the triangle (Fig. 1),
then
b sin A = h.
On replacing this in the right-hand side of equation 1), it becomes
sin B = h
a .
There are now three possibilities:
h
a < 1, which implies h < a (Fig. 1),
h
a = 1, which implies h = a (Fig. 2),
h
a > 1, which implies h > a (Fig. 3).
In the first of these -- h or b sin A < a -- there will be two triangles.
In the second -- h or b sin A = a -- there will be one right-angled triangle.
And in the third -- h or b sin A > a -- there will be no solution.
Tuesday, May 4, 2010
Study video
http://www.youtube.com/watch?v=Lsea3tMN99k
How to know if an equation is even, odd, or neither
. simple. but helpful . :)
What you need for your FINAL!
Each student will be allowed one sheet from twitter at the normal font size.
You will need a calculator for the final along with 2 pencils.
I will see you all in class....Good Luck!
Review
Law of sines
Law of cosines

(we did not learn this one in class but i thought it might be useful)
You can use herons formula if you are given all sides and no angles
where 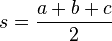
Review of the Ambiguous Case
|
|
By definition, the word ambiguous means open to two or more interpretations.
Such is the case for certain solutions when working with the Law of Sines.
| • If you are given two angles and one side (ASA or AAS), the Law of Sines will nicely provide you with ONE solution for a missing side. | |
 | • Unfortunately, the Law of Sines has a problem dealing with SSA. If you are given two sides and one angle (where you must find an angle), the Law of Sines could possibly provide you with one or more solutions, or even no solution. |
Before we investigate this situation, there are a few facts we need to remember.
Facts we need to remember: |  |
Let's look at some cases. In each example, decide whether the given information points to the existence of one triangle, two triangles or no triangles.
![]()
Example 1: In  , a = 20, c = 16, and m = 30º. How many distinct triangles can be drawn given these measurements?
, a = 20, c = 16, and m = 30º. How many distinct triangles can be drawn given these measurements?
 | Use the Law of Sines:
|
C = sin-1 (0.4) = 24º (to the nearest degree) - in Quadrant I.
Sine is also positive in Quadrant II. If we use the reference angle 24º in Quadrant II,
the angle C is 156º.
But, with m = 30º and m
Not possible!!!!
Therefore, m
![]()
Example 2: In  , a = 7, c = 16, and m = 30º. How many distinct triangles can be drawn given these measurements?
, a = 7, c = 16, and m = 30º. How many distinct triangles can be drawn given these measurements?
 | Use the Law of Sines:
|
Since sin C must be 1, no angle exists for angle C.
NO triangle exists for these measurements.
![]()
Example 3: In  , a = 10, b = 16, and m = 30º. How many distinct triangles can be drawn given these measurements?
, a = 10, b = 16, and m = 30º. How many distinct triangles can be drawn given these measurements?
 | Use the Law of Sines:
|
B = sin-1(.8) = 53.13010 = 53º.
Angles could be 30º, 53º, and 97º : sum 180º
The angle from Quadrant II could create angles 30º, 127º, and 23º : sum 180º
 |
|  |
This example is the Ambiguous Case. The information given is the postulate SSA (or ASS, the Donkey Theorem), but the two triangles that were created are clearly not congruent. We have two triangles with two sides and the non-included angle congruent, but the triangles are not congruent to each other.
COOOOOOOL STUFFFFFF
Astronomy and geography
 Trigonometric tables were created over two thousand years ago for computations in astronomy. The stars were thought to be fixed on a crystal sphere of great size, and that model was perfect for practical purposes. Only the planets moved on the sphere. (At the time there were seven recognized planets: Mercury, Venus, Mars, Jupiter, Saturn, the moon, and the sun. Those are the planets that we name our days of the week after. The earth wasn't yet considered to be a planet since it was the center of the universe, and the outer planets weren't discovered then.) The kind of trigonometry needed to understand positions on a sphere is called spherical trigonometry. Spherical trigonometry is rarely taught now since its job has been taken over by linear algebra. Nonetheless, one application of trigonometry is astronomy.
Trigonometric tables were created over two thousand years ago for computations in astronomy. The stars were thought to be fixed on a crystal sphere of great size, and that model was perfect for practical purposes. Only the planets moved on the sphere. (At the time there were seven recognized planets: Mercury, Venus, Mars, Jupiter, Saturn, the moon, and the sun. Those are the planets that we name our days of the week after. The earth wasn't yet considered to be a planet since it was the center of the universe, and the outer planets weren't discovered then.) The kind of trigonometry needed to understand positions on a sphere is called spherical trigonometry. Spherical trigonometry is rarely taught now since its job has been taken over by linear algebra. Nonetheless, one application of trigonometry is astronomy.  As the earth is also a sphere, trigonometry is used in geography and in navigation. Ptolemy (100-178) used trigonometry in his Geography and used trigonometric tables in his works. Columbus carried a copy of Regiomontanus' Ephemerides Astronomicae on his trips to the New World and used it to his advantage.
As the earth is also a sphere, trigonometry is used in geography and in navigation. Ptolemy (100-178) used trigonometry in his Geography and used trigonometric tables in his works. Columbus carried a copy of Regiomontanus' Ephemerides Astronomicae on his trips to the New World and used it to his advantage.
Engineering and physics
Although trigonometry was first applied to spheres, it has had greater application to planes. Surveyors have used trigonometry for centuries. Engineers, both military engineers and otherwise, have used trigonometry nearly as long.Physics lays heavy demands on trigonometry. Optics and statics are two early fields of physics that use trigonometry, but all branches of physics use trigonometry since trigonometry aids in understanding space. Related fields such as physical chemistry naturally use trig.
Review
Standard position
We say that an angle is in standard position when its vertex A is at the origin of the coordinate system, and its Initial side AB lies along the positive x-axis. We say that AB has "swept out" the angle BAC, and that AC is its Terminal side.

Coterminal angles
Angles are coterminal if, when in the standard position, they have the same terminal side.

Acute angle

Obtuse angle

Right angle

Reflex angle

A straight angle

A central angle is an angle For an inscribed angle Central Angle ![]()
![]()
![]()
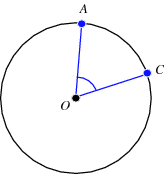
![]() with endpoints
with endpoints ![]() and
and ![]() located on a circle's circumference and vertex
located on a circle's circumference and vertex ![]() located at the circle's center . A central angle in a circle determines an arc
located at the circle's center . A central angle in a circle determines an arc ![]() .
.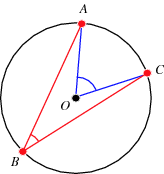
![]() and central angle
and central angle ![]() with the same endpoints,
with the same endpoints,![]()


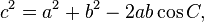


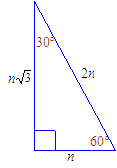
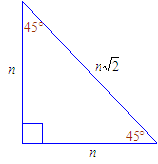
 .
. = positive decimal can lie in the first quadrant (acute <) or in the second quadrant (obtuse <).
= positive decimal can lie in the first quadrant (acute <) or in the second quadrant (obtuse <).





